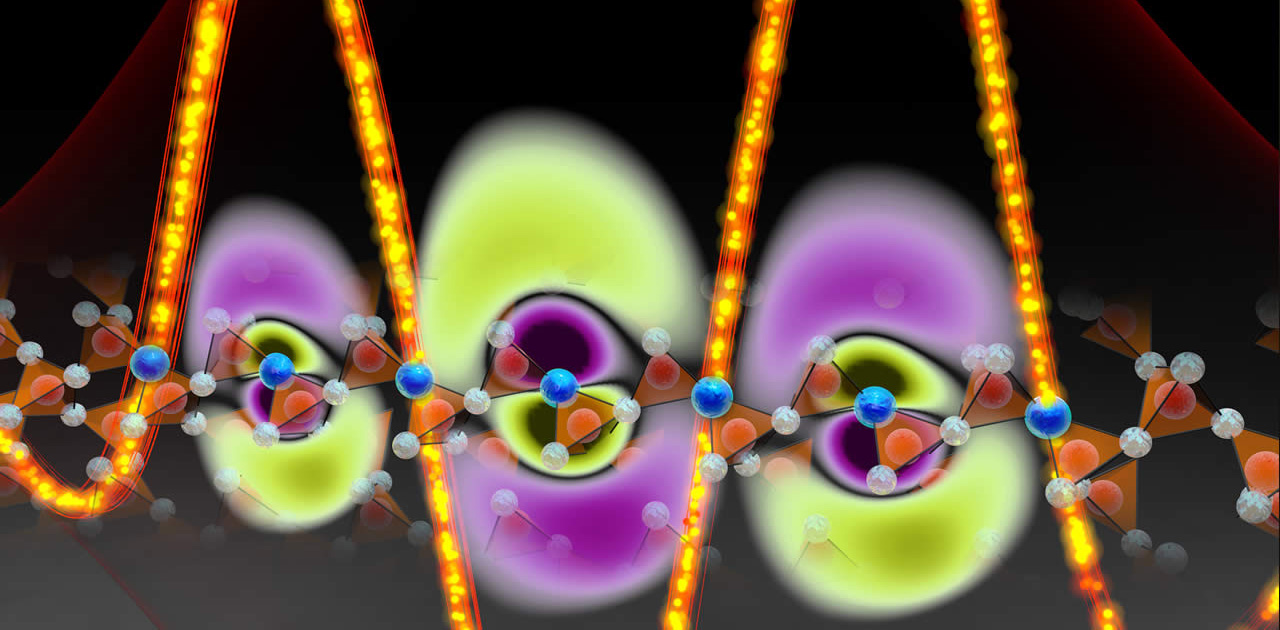
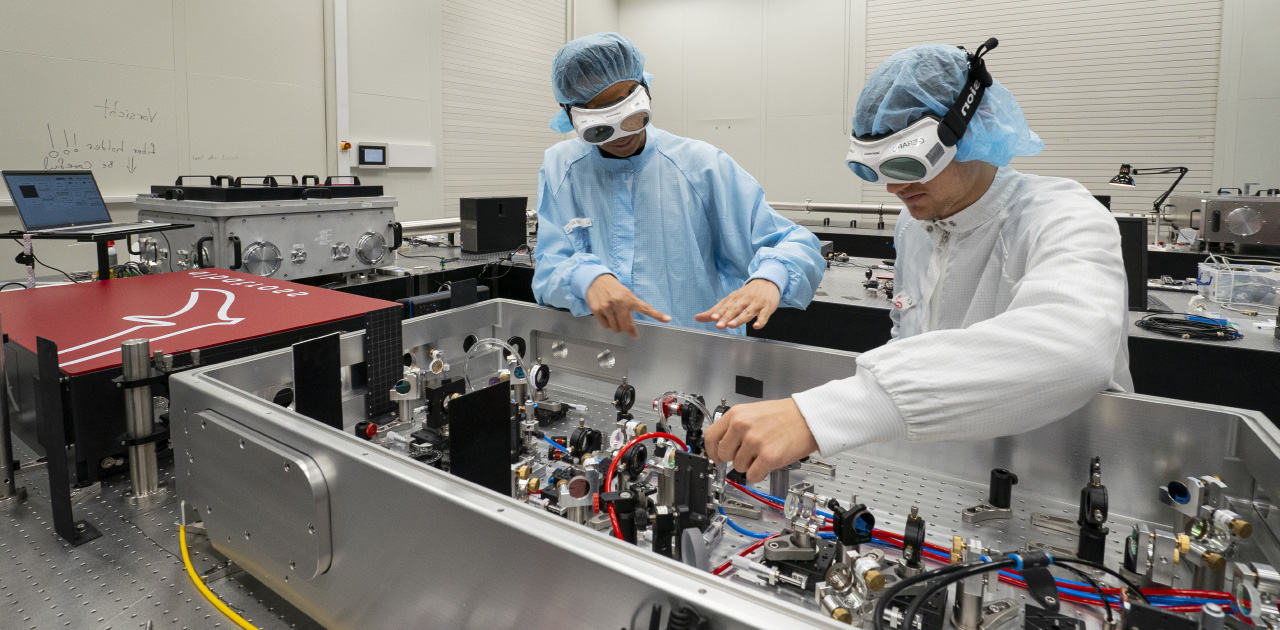
controlling and measuring ever faster electron dynamics with ever better accuracy is going to have applications across multiple disciplines
Research group of Dr. Nicholas Karpowicz & apl. Prof. Dr. Vladislav S. Yakovlev

Attosecond science has expanded access to microscopic processes, especially the motion of electrons. One exciting consequence is the possibility of forming a sub-femtosecond gate, providing a snapshot of the electromagnetic field faster than it can oscillate. We can directly record the waveform of light.
Why is that exciting? When a charged particle moves, its motion is inscribed into the electromagnetic field – reading the waveform lets us reconstruct it. So, not only can we record fields, we can use them observe processes that are too fast for traditional pump-probe spectroscopy.

see picture

signal control with light frequencies
We are extending these techniques to be not only time resolved, but also spatially-resolved: for example, an electric field microscope that records the full spatiotemporal properties of a light wave with sub-wavelength resolution.
To accelerate this line of attosecond research, we pursue both the scientific applications of field metrology and the tools and techniques that make it happen. A new line of research is based on highly-stable direct diode pumped Cr:ZnS oscillators to allow extremely precise studies of light matter interaction.
Finally, we develop open source software allowing our results to be easily reproduced, understood and extended by the wider community.


experimental tools & techniques
In order to replicate the precision and clarity with which conventional electronics can be characterized and understood, direct analogues for some of the fundamental tools in electronics were required:
Function generators: Before the measurement can begin, we need to be able to control the electric field that will interact with the target material. This field should be both repeatable and controllable; our laser sources provide just that capability. Using »phase-stable« sources, which provide trains of identical electric field waveforms, and field synthesis techniques to manipulate them into the forms best suited to the experiment, we exert precise control over the interactions we wish to study. Using multiple, high-energy sources in the visible, near-infrared, and mid-infrared spectral regions, plus nonlinear conversion to cover the ultraviolet and extreme ultraviolet, we are able to trigger and study dynamics across a wide spectrum of materials and types of interaction.
Oscilloscopes: Some of the most precise information on the interaction between light and matter is contained within the electromagnetic field. From Maxwell’s equations, we can see that the electric field is directly coupled to the motion of charged particles, allowing us to reconstruct the full history of the interaction. But how does one capture a laser field? That is, what can serve as an oscilloscope for infrared and visible radiation?
We make use of a set of techniques: attosecond streaking, electro-optic sampling as well as both linear and nonlinear polarization sampling.
attosecond streaking
This technique makes use of attosecond bursts of x-ray light as short as 70 attoseconds (SI units: as). Such a pulse can be used to directly measure petahertz laser fields inside an attosecond streak camera. This is done by directing the attosecond pulse onto a noble gas atom, causing an electron to be ejected via the photoelectric effect — the electron will emerge into the vacuum and travel to an electron spectrometer. If we apply a laser field to the atom at the same time as the attosecond pulse, the electron will be accelerated in the field, causing a shift of its final energy when it reaches the spectrometer. Thus, by precisely controlling when the attosecond pulse reaches the atom, we can sample the force applied by the laser to the electron and thereby map the electric field as a function of time.

see picture

streaking camera
electro-optic sampling
Made popular in the terahertz spectral domain, electro-optical sampling provides an alternative route to electric-field information, making use of nonlinear optical crystals to measure a laser field by its influence on the polarization of another laser pulse that simultaneously passes through the detection medium. Using ultrashort optical pulses, we are able to directly access the field oscillations of even near-infrared light.
photoconductive sampling
Using extremely short laser pulses to excite the active region of a small metal-dielectric-metal junction with extreme temporal precision, we can use solid-state optoelectronic devices to measure electric field transients based on the laser-field-induced generation of electric current. Driving these circuits with a longer-wavelength field provides access to the electric field waveform and gives new insight into the dynamics of charge currents switched at petahertz repetition rates.
nonlinear polarization sampling
Adopting a versatile scheme from the fields of microwave and terahertz spectroscopy, we can measure the laser-field waveform after passage through a nonlinear medium. This measurement is done twice. Once with the peak intensity of the laser set high enough to accumulate significant nonlinear modifications, and once at an intensity level low enough to ensure that the light-matter interaction is virtually free of nonlinearities. By comparison of the two recorded waveforms normalized for their difference in peak amplitude, we can extract the temporal evolution of the nonlinear polarization wave inside the sample. This allows for a sensitive quantitative investigation of the energy exchange dynamics between a laser field and a sample, the response time of the underlying nonlinearities and the electron kinetics responsible for the optical response.
attosecond diagnostics
Of course, different experiments demand different observations to provide a full physical picture. For this reason, we utilize three different attosecond beamlines for advanced experiments, including time-resolved photoelectron spectroscopy, x-ray and extreme ultraviolet time-resolved spectroscopy and more. Our toolbox includes electro-optical sampling and photoconductive switching at frequencies ranging from the microwave to near-infrared, photoelectron time-of-flight spectroscopy from gases and surfaces and attosecond transient absorption measurements in gaseous and solid systems. Nonlinear polarization sampling relies on the capability of a modified gas-phase photoelectron streaking set-up to sample the electric field of light.

see picture
attosecond photoemission of electrons

see picture

Headline: We employ a synergetic combination of experimental methods to explore electron dynamics in condensed-matter systems. Visible and X-Ray Absorption Spectroscopy probes the conduction band population, while photoelectron spectroscopy is sensitive to valence band occupation. Direct access to the light-field-driven carrier dynamics is achieved via attosecond polarization sampling and sub-cycle-resolved current metrology Photo: Thorsten Naeser
the FIDOPA project
In the FIDOPA (Field-Induction Decay enhanced via Optical Parametric Amplifier) group we aim to enhance, via optical nonlinear phenomena, the sensitivity and optical bandwidth of electric-field resolved measurement of mid-infrared light pulses. Specifically, we are developing a novel technique, based on an optical parametric amplifier, to boost the free-induction-decay signal from the molecular response by over 100 times. Together with the electro-optic sampling technique used for electric-field-resolved molecular fingerprinting, the system could simultaneously achieve high sensitivity and broad spectral reach for infrared spectroscopy. Applying this to biofluids, our ambition is to uncover the slightest changes in the molecular composition of blood samples for detecting illnesses before they manifest.

see picture

Electro-optic sampling via the Pockels effect

see picture

inspecting the electro-optic sampler
theoretical tools & techniques
Theoretical research in our group has several purposes: explaining measured data, providing a theoretical basis for planning future measurement campaigns, as well as identifying new promising research directions. For all these purposes, our main tool is a computational experiment, where we use various models and approximations to deepen our understanding of processes that accompany the interaction of extremely short light pulses with condensed matter. There is no single theoretical model that would adequately describe all the relevant processes, and we often learn important lessons by comparing outcomes from different models.
time-dependent density functional theory
In this approximation, every electron interacts with other electrons via a time-dependent mean- field potential, which depends on the electron density. Even though this approach is limited in its ability to account for electron-electron collisions and correlation, it is currently the most advanced approach that can be used to model highly nonlinear interaction of intense laser pulses with solids. Employing more sophisticated methods is prohibited by the fact that such a pulse typically populates tens of energy bands in the entire Brillouin zone with a final state that requires thousands of crystal momenta to discretize reciprocal space properly. Even though very good agreement between experiment and time-dependent density functional theory was demonstrated, this approach has drawbacks: calculations require significant computational resources (hundreds of core hours), results are sensitive to the choice of an exchange-correlation potential, and accounting for decoherence is not straightforward within this approach.
semiconductor Bloch equations
In its general formulation, this is a many-body approach that is capable of describing electron- electron and electron-phonon interactions from first principles. Such a general description, however, would require the employment of many-particle density matrices, rendering simulations infeasible in the context of strong-field light-matter interaction. As long as the state of a many- electron quantum system is approximated with single-particle density matrices, the capability of this approach to account for correlation effects is rather limited. Nevertheless, semiconductor Bloch equations have an advantage over methods that operate with single-electron wave functions: density matrices make it easy to account for electron-phonon interaction and electron-electron correlation via semi-phenomenological relaxation terms.
time-dependent Schrödinger equation for independent particles
In those cases where a laser pulse is so short that relaxation plays no significant role during light-matter interaction, relaxation terms in semiconductor Bloch equations can be dropped. If, at the same time, an external electric field negligibly changes the average Coulomb interaction between electrons, a system of time-dependent Schrödinger equations adequately describes electron dynamics. In this independent-particle approach, electrons do not explicitly interact with each other, except via a macroscopic electromagnetic field that their motion may induce. In spite of this, this method does not neglect the electron-electron interaction entirely. This interaction enters the model via a stationary basis of Bloch states that we obtain from ab initio calculations, which do account for the interaction between electrons. By comparing outcomes of such simulations to those of the time-dependent density functional theory, we often see a very good agreement. The main advantage of working in a stationary basis of Bloch states is the flexibility of this approach: input data (band energies and matrix elements) can be obtained with any DFT code or even with post-DFT methods, such as quasiparticle self-consistent GW. Also, solving a system of time-dependent Schrödinger equations in a stationary basis is much faster than using time-dependent density functional theory or solving semiconductor Bloch equations. For this reason, we use this method often, and we have developed a python code for such calculations. In this code, the most time-consuming functions are parallelized using Numba, and the code is publicly available via GitHub
A mobile version for attoworld.de is under construction.